-
 Leontiskos
3.8kand when the OP is in the Logic sub-forum it makes sense to default to trained logic — Moliere
Leontiskos
3.8kand when the OP is in the Logic sub-forum it makes sense to default to trained logic — Moliere
@Janus' point applies to logic as well. Formal logic is parasitic on natural logic, and "logic" does not mean "formal logic," or some system of formal logic. A lot of folks around here get into trouble because they can't see beyond their own logical system. Tones even mistakes natural language for his own system, and normatively interprets natural language in terms of his system.
And to read Flannel Jesus' posts is to realize that he did not intend the OP in any special sense. I see no evidence that he was specifically speaking about material implication. -
 Moliere
5.1kAnd to read Flannel Jesus' posts is to realize that he did not intend the OP in any special sense. I see no evidence that he was specifically speaking about material implication. — Leontiskos
Moliere
5.1kAnd to read Flannel Jesus' posts is to realize that he did not intend the OP in any special sense. I see no evidence that he was specifically speaking about material implication. — Leontiskos
Yeah, looking at OP at least, I can see how there's ambiguity there: whether material implication, or some other meaning, was meant isn't specified in the OP and so whatever meaning was meant there's still ambiguity there (which may explain some of the divergence here that I'm surprised to find)
The part where "A" is used as a variable is what made me jump to propositional logic.
Your points about the difference between two versions of contradiction was interesting and I was thinking about it then got sidetracked in reading the back-and-forth.
Formal logic is parasitic on natural logic, and "logic" does not mean "formal logic," or some system of formal logic. — Leontiskos
Yeah, we agree there. I think @TonesInDeepFreeze does too, given the various caveats they gave in their posts about different forms of logic.
And again we come back to: as long as the people doing philosophy stipulate definitions they agree :D -
 Leontiskos
3.8k- Yes, I think this is right.
Leontiskos
3.8k- Yes, I think this is right.
I keep thinking about my aversion to "∴ ~A" ().
The most basic objection is that an argument with two conditional premises should not be able to draw a simple or singular conclusion (because there is no simple claim among the premises).
I had not been following the line in the thread stemming from your claim, but 's proof fortuitously gave me an inroad, and his proof has nothing in particular to do with material implication.
So then we have (p→(q∧¬q)), and I think your question immediately arises:
Can anyone think up a real world example where you would point out that A implies both B and not-B except for saying something along the lines of:
"A implies B and not-B, therefore clearly not-A." — Count Timothy von Icarus
But I would press further and wonder whether we ever do say things along those lines, in a strict sense:
The way you would usually use it in any sort natural language statement would be to say: "Look, A implies both B and not-B, so clearly A cannot be true." You don't have a contradiction if you reject A, only if you affirm it.
This is a fairly common sort of argument. Something like: "if everything Tucker Carlson says about Joe Biden is true then it implies that Joe Biden is both demented/mentally incompetent and a criminal mastermind running a crime family (i.e., incompetent and competent, not-B and B) therefore he must be wrong somewhere." — Count Timothy von Icarus
This actually runs head-on into the problem that I spelled out <here>. Your consequent is simply not a contradiction in the sense that gave (i.e. the second clear sense of "contradiction" operating in the thread). I don't think (p→(q∧¬q)) ever occurs (in reality). This is obviously related to @Janus' critique. I want to say, "Yes, if that proposition were true, then ¬p would follow, but it is never true." Hence Lionino's point, which is elementary but essential:
...and "imply ¬A" as [meaning] the proposition being True means A is False. — Lionino
Which goes back to a central question. How are we interpreting the OP? In my sense or in Moliere's sense?
...I should also note that Tones gave an argument for ~A in which he attempted to prove it directly, without going through Lionino's equivalence proof. This is an acceptable argument by basic logical standards, but I have always had difficulty with argument by supposition. What does it mean to suppose A and then show that ~A follows? This gets into the nature of supposition, how it relates to assertion, and the LEM. It also gets into the difference between a reductio and a proof proper. The point is one I had already made in a post that Tones was responding to, "You think the two propositions logically imply ~A? It seems rather that what they imply is that A cannot be asserted" ().
Still, as I conceded to Lionino, I think his equivalence proof suffices to show that we can draw ~A if the proposition is true. It just seems that it is never true. -
 Leontiskos
3.8kYeah, looking at OP at least, I can see how there's ambiguity there: whether material implication, or some other meaning, was meant isn't specified in the OP and so whatever meaning was meant there's still ambiguity there (which may explain some of the divergence here that I'm surprised to find) — Moliere
Leontiskos
3.8kYeah, looking at OP at least, I can see how there's ambiguity there: whether material implication, or some other meaning, was meant isn't specified in the OP and so whatever meaning was meant there's still ambiguity there (which may explain some of the divergence here that I'm surprised to find) — Moliere
Right, and note also the way that Flannel confuses the conditions of a material implication with the principle of explosion beginning <here>.
The part where "A" is used as a variable is what made me jump to propositional logic. — Moliere
I gave an example of using "A" without material implication earlier, "Supposing A, would B follow?" ().
Your points about the difference between two versions of contradiction was interesting and I was thinking about it then got sidetracked in reading the back-and-forth. — Moliere
I think that is a central point, which Lionino was the first to make explicit on the first page of the thread. It goes back to the uncertainty of the asterisk in my first post, as no one has set out the exact way that the two versions relate. The simple account, which I have set out, is that to contradict is to negate, and what it means to negate depends on one's logical context. Tones was assuming a truth-functional context where negation is the reversal of the truth table. -
 Leontiskos
3.8kYour points about the difference between two versions of contradiction was interesting and I was thinking about it then got sidetracked in reading the back-and-forth. — Moliere
Leontiskos
3.8kYour points about the difference between two versions of contradiction was interesting and I was thinking about it then got sidetracked in reading the back-and-forth. — Moliere
The original question was, "Do (A implies B) and (A implies notB) contradict each other?"
On natural language they contradict each other.
On the understanding of contradiction that I gave in the first post, they do not contradict each other, and their conjunction is not a contradiction.
On the understanding of contradiction that you gave in the second post, their conjunction is not a contradiction, but their conjunction does contain a contradiction (as showed).
It is that contradiction contained within the conjunction that bubbles up and creates all of the strangeness, and it is worth noting that this contradiction is a direct result of the idiosyncrasies of material implication; they are only logically consistent on account of material implication. It has been some time since I studied formal logic, but I would want to say something along the lines of this, "A proposition containing (p∧¬p) is not well formed." Similar to what I said earlier, "When we talk about contradiction there is a cleavage, insofar as it cannot strictly speaking be captured by logic. It is a violation of logic" (). My idea would be that (p∧¬p) is outside the domain of the logic at hand, and to try to use the logic at hand to manipulate it results in paradoxes.
But I'm sure others have said this better than I, and the principle of explosion is in fact relevant here insofar as it too relies on the incorporation of a contradiction into the interior logical flow of arguments.
6 pages too many on this thread. — Lionino
Perhaps. :lol: -
 Leontiskos
3.8k((a→b)∧(a→¬b))↔¬a is valid — Lionino
Leontiskos
3.8k((a→b)∧(a→¬b))↔¬a is valid — Lionino
My point is that it is a vacuous instance of validity, more clearly seen in the form <((a→(b∧¬b))↔¬a>. It is formal logic pretending to say something. As I claimed above, there is no actual use case for such a proposition, and I want to say that propositions which contain (b∧¬b) are not well formed. They lead to an exaggerated form of the problems that has referenced. We can argue about material implication, but it has its uses. I don't think propositions which contain contradictions have their uses.
This is perhaps a difference over what logic is. Is it the art of reasoning and an aid to thought, or just the manipulation of symbols? I would contend that one reason we know it is not merely the manipulation of symbols is because the rules are not arbitrary, and I am proposing the well-formed-formula rule as yet another non-arbitrary rule. Unless I am wrong and there is some good reason we need to allow for propositions to contain internal contradictions?
I am concerned that logicians too often let the tail wag the dog. The ones I have in mind are good at manipulating symbols, but they have no way of knowing when their logic machine is working and when it is not. They take it on faith that it is always working and they outsource their thinking to it without remainder. -
 Leontiskos
3.8kI could try to make the critique more precise, although the only person on these forums who has shown a real interest in what I would call 'meta-logic' is .
Leontiskos
3.8kI could try to make the critique more precise, although the only person on these forums who has shown a real interest in what I would call 'meta-logic' is .
Every time we make an inference on the basis of a contradiction a metabasis eis allo genos occurs (i.e. the sphere of discourse shifts in such a way that the demonstrative validity of the inference is precluded). Usually inferences made on the basis of a contradiction are not made on the basis of a contradiction “contained within the interior logical flow” of an argument. Or in other words, the metabasis is usually acknowledged to be a metabasis. As an example, when we posit some claim and then show that a contradiction would follow, we treat that contradiction as an outer bound on the logical system. We do not incorporate it into the inferential structure and continue arguing. Hence the fact that it is a special kind of move when we say, “Contradiction; Reject the supposition.” In a formal sense this move aims to ferret out an inconsistency, but however it is conceived, it ends up going beyond the internal workings of the inferential system (i.e. it is a form of metabasis).
Now suppose we draw out the argument for ¬A:
- ((A→(B∧¬B))
- ∴ ¬A
This is a covert metabasis. It is a metabasis that is not acknowledged to be a metabasis. This has to do with the contradiction, (B∧¬B), which is interpreted equivocally as both a proposition and a truth value (“false”). The difference between a truth value and a proposition is flubbed because what is posited is purely formal, and can never exist in reality (i.e. a contradiction). In order to affirm such a proposition as being true, we must affirm something which could never actually be affirmed, and thus the formal logic here parts ways with reality in a drastic manner. Normal logical propositions do not contain contradictions, and therefore do not require us to do such strange things!
You could also put this a different way and say that while the propositions ((A→(B∧¬B)) and (B∧¬B) have truth tables, they have no meaning. They are not logically coherent in a way that goes beyond mere symbol manipulation. We have no idea what (B∧¬B) could ever be expected to mean. We just think of it, and reify it as, "false" - a kind of falsity incarnate.* Is this then a critique of truth functionality? Maybe, but I want to say that truth functionality can have value where contradictions are not allowed.
* A parallel equivocation occurs here on 'false' and 'absurd' or 'contradictory'. Usually when we say 'false' we mean, "It could be true but it's not." In this case it could never be true. It is the opposite of a tautology—an absurdity or a contradiction.
-
Edit:
We can apply Aristotelian syllogistic to diagnose the way that the modus tollens is being applied in the enthymeme:
- ((A→(B∧¬B))
- ∴ ¬A
Viz.:
- Any consequent which is false proves the antecedent
- (B∧¬B) is a consequent which is false
- ∴ (B∧¬B) proves the antecedent
In this case the middle term is not univocal. It is analogical (i.e. it posses analogical equivocity). Therefore a metabasis is occurring. As I said earlier:
* A parallel equivocation occurs here on 'false' and 'absurd' or 'contradictory'. Usually when we say 'false' we mean, "It could be true but it's not." In this case it could never be true. It is the opposite of a tautology—an absurdity or a contradiction. — Leontiskos
Now one could argue for the analogical middle term, but the point is that in this case we are taking modus tollens into new territory. Modus tollens is based on the more restricted sense of 'false', and this alternative sense is a unfamiliar to modus tollens. This is a bit like putting ethanol fuel in your gasoline engine and hoping that it still runs.
Note that the (analogical) equivocity of 'false' flows into the inferential structure, and we could connote this with scare quotes. (B∧¬B) is "false" and therefore the conclusion is "implied." The argument is "valid." -
 Count Timothy von Icarus
3.2k
Count Timothy von Icarus
3.2k
Let A = "Unenlightened's testimony is unreliable"
Let B = "Unenlightened tells the truth"
not B ="Unenlightened does not tell the truth"
↪Count Timothy von Icarus might note that ↪unenlightened's testimony is reliable
That's a cute one. It seems to trade off the ambiguity of translating statements into logic. Obviously when we say someone's testimony is "unreliable" what we mean is that some of their statements are true and some are not true. But there is nothing contradictory about A implying some B are C and A also implying that some B are not C, and so we won't be forced to deny A.
I think one of the challenges inherit with formal logic is that even fairly straightforward arguments of the sort you might find on some science blog or op-ed end up requiring all sorts of stuff to formally model correctly: counterfactuals, modality, temporality, etc. It gets very complex very quickly.
It's even hard with comically bad arguments like the pic below. Premises like "solar panels reflect more heat than grass or trees," and "small changes in ground temperature can cascade into large changes in weather systems, including tornado formation," are all true, but the conclusion that solar farms are "tornado incubators" is still baseless.
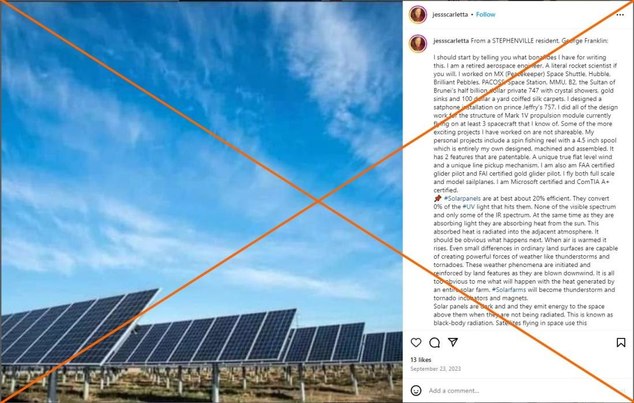
-
 Count Timothy von Icarus
3.2kA = There are vampires.
Count Timothy von Icarus
3.2kA = There are vampires.
B = Vampires are dead.
Not-B = Vampires are living.
As you can clearly judge, this truth table works with Ts straight across the top, since vampires are members of the "living dead." Fools who think logic forces them to affirm ~A are like to end up missing all their blood.
You might be interested in relevance logic, which tries to deal with the paradoxes of material implication: https://plato.stanford.edu/entries/logic-relevance/
There is a section in Tractatus where Wittgenstein declares that belief in a causal nexus is a "superstition" and holds up logical implication as sort of the "real deal." I think this is absolutely backwards. We come up with the idea of logical implication from experience, from the way the world works. This is mistaking an abstraction for reality, and this is ultimately where I think the discomfort with the paradoxes come from.
There is some interesting stuff on modeling relevance logic in terms of information theory I've seen but I forget where I found it. -
 Leontiskos
3.8k- I went back to read this. I agree with the conclusion:
Leontiskos
3.8k- I went back to read this. I agree with the conclusion:
...Logicians have an answer to the above charge, and the answer is perfectly tight and logically consistent. That is part of the problem! Consistency is not enough. Logic should be not just a mathematically consistent system but a human instrument for understanding reality, for dealing with real people and things and real arguments about the real world. That is the basic assumption of
the old logic.
But I think Kreeft is working with a caricature in the earlier parts, as he has a tendency to do:
...The conclusion ("Lassie is a dog") may be true, but it has not been proved by this argument. It does not "follow" from the premises.
Now in Aristotelian logic, a true conclusion logically follows from, or is proved by, or is "implied" by, or is validly inferred from, only some premises and not others. The above argument about Lassie is not a valid argument according to Aristotelian logic. Its premises do not prove its conclusion. And common sense, or our innate logical sense, agrees. However, modern symbolic logic disagrees. One of its principles is that "if a statement is true, then that statement is implied by any statement whatever."
He is falling into equivocation between validity and material implication. Modern logic agrees with Aristotelian logic in saying that, "It does not follow from the premises." That a material conditional is true does not mean that the consequent can be drawn, and if Kreeft tries to draw the consequent when the antecedent is false, the modern logician will rightly accuse him of failing to respect the conditions of modus ponens. I think Kreeft is involved in word games here, but in his defense he might say that the modern logician is involved in word games with his word "implies."
This principle is often called "the paradox of material implication." Ironically, "material implication" means exactly the opposite of what it seems to mean. It means that the matter, or content, of a statement is totally irrelevant to its logically implying or being implied by other statements.
Oh, Kreeft knows full well that "material" is contrasted with "formal," and that content is formal, not material. :roll:
Logician: So, class, you see, if you begin with a false premise, anything follows.
Student: I just can't understand that.
Logician: Are you sure you don't understand that?
Student: If I understand that, I'm a monkey's uncle.
Logician: My point exactly. (Snickers.)
Student: What's so funny?
Logician: You just can't understand that.
:grin:
The relationship between a premise and a conclusion is called "implication," and the process of reasoning from the premise to the conclusion is called "inference" In symbolic logic, the relation of implication is called "a tnith-functional connective," which means that the only factor that makes the inference valid or invalid, the only thing that makes it true or false to say that the premise or premises validly imply the conclusion, is not at all dependent on the content or matter of any of those propositions, but only whether the premise or premises are true or false and whether the conclusion is true or false.
I agree that material implication has problems, but if you want a tidy, "algorithmic" system, then these sorts of problems are inevitable. -
 Count Timothy von Icarus
3.2k
Count Timothy von Icarus
3.2k
The conservation part was the only reason I remembered it TBH.
I agree that material implication has problems, but if you want a tidy, "algorithmic" system, then these sorts of problems are inevitable.
Or one that isn't horrifically complex. I actually think that is what gets people more than the "paradoxes of implication." People can learn that sort of thing quite easily. What seems more confusing is the way in which fairly straightforward natural language arguments can end up requiring a dazzling amount of complexity to model. -
 Leontiskos
3.8kYou might be interested in relevance logic, which tries to deal with the paradoxes of material implication: https://plato.stanford.edu/entries/logic-relevance/ — Count Timothy von Icarus
Leontiskos
3.8kYou might be interested in relevance logic, which tries to deal with the paradoxes of material implication: https://plato.stanford.edu/entries/logic-relevance/ — Count Timothy von Icarus
Thanks, I may check this out in time. My sense, though, is that you can't fully formalize reasoning. In particular Aristotle's final condition for demonstrative knowledge at 71b22 of the Posterior Analytics is something that I think can never be fully formally modeled, "[demonstrative understanding depends on things that are...] explanatory (aitia) of the conclusion." To understand why something is the way it is requires more than symbol-manipulation, and to understand why B follows from A requires understanding why B is the way it is.
There is a section in Tractatus where Wittgenstein declares that belief in a causal nexus is a "superstition" and holds up logical implication as sort of the "real deal." I think this is absolutely backwards. — Count Timothy von Icarus
That sounds like classic Humean backwards-reasoning. :lol:
In that very same paragraph of Posterior Analytics Aristotle points out that in order for an argument to produce knowledge the premises must be better-known than the conclusion, which strikes me as indicative Hume's significant error. Hume takes premises that are very implausible and leverages them to disprove beliefs that are highly plausible, and this is also why his arguments are rightly dismissed even by those who cannot offer a point-for-point refutation.
Or one that isn't horrifically complex. I actually think that is what gets people more than the "paradoxes of implication." People can learn that sort of thing quite easily. What seems more confusing is the way in which fairly straightforward natural language arguments can end up requiring a dazzling amount of complexity to model. — Count Timothy von Icarus
Right. -
 Janus
16.8k
Janus
16.8k
Some interesting points from both of you, so for me not "six pages too many".
Do (A implies B) and (A implies notB) contradict each other? — flannel jesus
I woke in the middle of the night and realized there is an alternative interpretation of the above in natural language. I remain convinced that reading the two propositions as "B follows from A" and "B does not follow from A" means that they contradict one another.
However, reading (A implies notB) as "something other than B (caveat: also) follows from A". would be consistent with "B follows from A", because it would not deny that B also follows from A.
That's my kindergarten contribution for what it's worth. -
 Leontiskos
3.8kI woke in the middle of the night and realized there is an alternative interpretation of the above in natural language. I remain convinced that reading the two propositions as "B follows from A" and "B does not follow from A" means that they contradict one another. — Janus
Leontiskos
3.8kI woke in the middle of the night and realized there is an alternative interpretation of the above in natural language. I remain convinced that reading the two propositions as "B follows from A" and "B does not follow from A" means that they contradict one another. — Janus
That makes sense to me (even though symbolic logicians must interpret all such things as material implication, as they have no alternative). Related:
Yet in natural language when we contradict or negate such a claim, we are in fact saying, "If lizards were purple, they would not be smarter." We say, "No, they would not (be smarter in that case)." The negation must depend on the sense of the proposition, and in actuality the sense of real life propositions is never the sense given by material implication. — LeontiskosJanus' point about natural language is something like this:
Supposing A, would B follow?
Bob: Yes
Sue: No
Now Sue has contradicted Bob. The question is, "What has Sue claimed?" — Leontiskos
It seems to me that it is key to understand that, "The negation must depend on the sense of the proposition..." Material implication is the way it is for much the same reason that humans are the way they are given Epimetheus' mistake. When the logic gods got around to fashioning material implication they basically said, "Well if the antecedent is true and the consequent is true then obviously the implication is true, and if the antecedent is true and the consequent is false then obviously the implication is false, but what happens in the other cases?" "Shit! We only have 'true' and 'false' to work with! I guess we just call it 'true'...?" "Yeah, we certainly can't call it 'false'."
I haven't thought about this problem in some time, but last time I did I decided that calling the vacuous cases of the material conditional 'true' is like dross. In a tertiary logic perhaps they would be neither true nor false, but in a binary logic they must be either true or false, and given the nature of modus ponens and modus tollens 'true' works much better. It's a bit of a convenient fiction. This is not to say that there aren't inherent problems with trying to cast implication as truth-functional, but it seems to me that an additional problem is the bivalence of the paradigm.
Yet some here have interpreted the OP in such a way that this 'dross' gets repackaged and marketed as gold. The undesirable behavior of material implication is basically supposed to be ignored, not utilized for logic parlor tricks.
That's my kindergarten contribution for what it's worth. — Janus
I think your basic intuition is correct. It resists the crucial methodological error of "trusting the logic machine to the extent that we have no way of knowing when it is working and when it is not" (). We need to be able and willing to question the logic tools that we have built. If the tools do not fit reality, that's a problem with the tools, not with reality (). -
 flannel jesus
2.1kHowever, reading (A implies notB) as "something other than B (caveat: also) follows from A". would be consistent with "B follows from A", because it would not deny that B also follows from A. — Janus
flannel jesus
2.1kHowever, reading (A implies notB) as "something other than B (caveat: also) follows from A". would be consistent with "B follows from A", because it would not deny that B also follows from A. — Janus
Yeah that's a good explanation for why it intuitively makes sense that they're a contradiction.
Consider this as an intuitive explanation for why they aren't a contradiction:
A implies B can be rephrased as (not A or B)
A implies not B can be rephrased as (not A or not B)
Do you think (not A or B) and (not A or not B) contradict? -
 javra
2.7kYou could also put this a different way and say that while the propositions ((A→(B∧¬B)) and (B∧¬B) have truth tables, they have no meaning. They are not logically coherent in a way that goes beyond mere symbol manipulation. We have no idea what (B∧¬B) could ever be expected to mean. We just think of it, and reify it as, "false" - a kind of falsity incarnate.* — Leontiskos
javra
2.7kYou could also put this a different way and say that while the propositions ((A→(B∧¬B)) and (B∧¬B) have truth tables, they have no meaning. They are not logically coherent in a way that goes beyond mere symbol manipulation. We have no idea what (B∧¬B) could ever be expected to mean. We just think of it, and reify it as, "false" - a kind of falsity incarnate.* — Leontiskos
Here’s a possible real-world example (which I think is common knowledge in some circles, though I don’t now recall where I picked up the example from):
A = a cat is sleeping outstretched on the threshold of the entry door to a house.
B = the cat is in the house
notB = the cat is not in the house
In this example, how does A not imply both B and notB in equal measure?
It so far seems to me that one can then affirm that, due to A, both B and notB are equally true. Furthermore, the just specified can then be affirmed with the same validity as affirming that A implies both B and notB to be equally false.
This, however, would still not be a contradiction, for while both B and notB occur (else don’t occur) at the same time as a necessary consequence of A, they nevertheless both occur (else don’t occur) in different respects.
As I so far see things, this addresses the principle of the excluded middle. But the fault would then not be with this principle of itself but, instead, with faulty conceptualizations regarding the collectively exhaustive possibilities in respect to what happens to in fact be the actual state of affairs.
This same type of reasoning can then be further deemed applicable to well enough known statements such as “neither is there a self nor is there not a self”. This latter proposition would be contradictory only were both the proposition’s clauses to simultaneously occur in the exact same respect. Otherwise, no contradiction is entailed by the affirmation.
Asking this thinking I (as a novice when it comes to formal modern logics) might have something to learn from any corrections to the just articulated. -
 Leontiskos
3.8kA = a cat is sleeping outstretched on the threshold of the entry door to a house.
Leontiskos
3.8kA = a cat is sleeping outstretched on the threshold of the entry door to a house.
B = the cat is in the house
notB = the cat is not in the house
In this example, how does A not imply both B and notB in equal measure? — javra
Yes, this is similar to 's vampire argument.
As I so far see things, this addresses the principle of the excluded middle. But the fault would then not be with this principle of itself but, instead, with faulty conceptualizations regarding the collectively exhaustive possibilities in respect to what happens to in fact be the actual state of affairs. — javra
Right: another way of putting it is that you are expressing a phenomenon which is not able to be captured by the logic at hand.
This same type of reasoning can then be further deemed applicable to well enough known statements such as “neither is there a self nor is there not a self”. — javra
This reminds me of the reasoning and forms of denial that both Buddhists and Pyrrhonic Skeptics developed, for similar reasons.
This latter proposition would be contradictory only were both the proposition’s clauses to simultaneously occur in the exact same respect. Otherwise, no contradiction is entailed by the affirmation. — javra
Right: if the terms of the argument are equivocal then the conclusion does not follow, and in this case the conclusion is the claim that a contradiction is occurring.
Asking this thinking I (as a novice when it comes to formal modern logics) might have something to learn from any corrections to the just articulated. — javra
It seems accurate to me. I see it as a heavier critique of bivalent logic than the one I was trying to give, but there are a lot of similarities.
Presumably the objection would be something like, "If you hear dogs barking outside and your roommate asks you if the cat is in the house, isn't he asking a question with only two mutually exclusive answers?" Or in other words, I think someone like Wittgenstein might say that the sense of "in the house" should be traced back to the purposes of the speaker, and that where the purposes are unclear the answer will also be unclear. If "in the house" means that the cat is locked inside and safe, then there is no ambiguity. I think there is some merit to this objection, but my guess is that there are nevertheless phenomena which bivalent logic struggles with. There are a lot of ways you could go with this sort of topic, and I am not well versed on polyvalent logic.
-
Edit: I sort of forgot to address the way your post interacts with the thing you were quoting. When a symbolic logician writes out (B∧¬B), they are not thinking of something like, "Yes and no," where the 'yes' applies to one aspect or consideration of the question and 'no' applies to a different aspect or consideration of the question. They are thinking of a formal contradiction, where something is and is not in precisely the same way. It is this formal contradiction which justifies their modus tollens and the affirmation of ¬A. If they are talking about something like your cat then as you rightly say the inferences that they depend on (and that I oppose) are no longer available for use. In that case I have no objection, for what I was objecting to has disappeared.
Note, though, that this problem does not seem to go away on polyvalent logic. It is still possible to syntactically represent an absurdity like (B∧¬B) in more complex logics, such as Buddhist logic. It's just more difficult to do.
The other relevant matter is whether we are thinking about speakers or whether we are thinking about propositions in the abstract. There are strong arguments for the idea that a conclusion requires at least two premises, and although the argument I am taking issue with is arguably an enthymeme (with a hidden premise), there is still a strange way in which the conclusion has only one premise insofar as nothing additional needs to be independently affirmed. This moves quickly away from a speaker-conception idea of propositions and logic, as if arguments could be self-proving, with no middle term. What is at stake, then, is not a logical inference in the psychological sense but rather a formal identity between the truth tables of two propositions. It is a case where the "inference" is based on nothing more than the regrettable idiosyncrasies of the logical system, and that could never be made by those who are not privy to the arbitrary conventions of the system. -
 Leontiskos
3.8kIt has been some time since I studied formal logic, but I would want to say something along the lines of this, "A proposition containing (p∧¬p) is not well formed." — Leontiskos
Leontiskos
3.8kIt has been some time since I studied formal logic, but I would want to say something along the lines of this, "A proposition containing (p∧¬p) is not well formed." — Leontiskos
The obvious objection to this idea is to note that this restriction goes beyond the typical syntactical requirements for a formula being well formed. I do see this, and it is possible that in rejecting this I am doing irreparable damage to modern symbolic logic. Perhaps my idea is that if someone engages in these sorts of inferences then there should be added an asterisk to their conclusion on account of the fact that this form of metabasis is highly questionable. I mostly want attention to be paid to what we are doing, and to be aware of when we are doing strange things. -
 javra
2.7kYes, this is similar to ↪Count Timothy von Icarus
javra
2.7kYes, this is similar to ↪Count Timothy von Icarus
's vampire argument. — Leontiskos
Yes, I forgot to give Count Timothy a mention. Thanks for pointing that out. I thought the example of the cat to be more “real-world” than that of vampires in that it is something that can physically happen in our world, and most likely has. But yes, it’s the same general issue in respect to logic.
As to polyvalent systems of logic, I’m one to find the notion of “partial truths” to be quite applicable to the world we live in. One of the most immediate and commonplace examples of this can be that of what one is seeing at any moment M. We generally say, “I am looking at …” to implicitly specify what we are willfully focusing on visually. But to specify what we are seeing is a quite different matter; such as, for example, were a judge to ask one “what did you see that night?” In such a context, it is literally impossible to give a “full truth” regarding what one saw or else sees: from the issue of needing to describe everything one is aware of occurring within one’s peripheral vision to that of needing to describe all the minute details of what one witnesses in one’s focal point of vision. A book would be required for this, and even then the telling would still be incomplete. Partial truths, such as that of what we are seeing, could then be contrasted with each other for degrees of fullness; such that what results, as one example among others, is the comparative attributes of some proposition being more true (or truthful), else most true, by comparison to some other proposition (and this without necessitating any falsehoods being expressed). In contrast, I find that falsehoods in the form of lies will always contain some (at least background) truths in order to be in any way believed by those to whom they're told. And in all this I find extensive interest. But maybe all this talk of partial truths is too far afield for the current thread. Although it certainly entwines quite well with most if not all systems of polyvalent logic.
All the same, thank you for the thoughtful reply, the "edit" portion of it included. Yes, my other post regarding "yes and no" was poorly formulated for the context; other such possible answers can include "it is and it isn't" and "they're the same but different" (which I do find interest in as pseudo-contradictions of sorts); but yes, they don't quite address implications. -
 Count Timothy von Icarus
3.2k
Count Timothy von Icarus
3.2k
I think issues like the cat are simply mistranslations and over simplifications. The statement should be something like:
The cat is sitting across the threshold of the house, therefore some of the cat is in the house and some of the cat is in the house. This is not contradictory. The same issue is in play here: .
This seems more like a case of "user error." It's like you cannot blame a coding language for a programmer writing code that correctly instructs it to do the wrong thing. The paradoxes of material implication go beyond this sort of misuse though.
If you affirm A there is a straightforward contradiction implied (B and not-B). But the statement as a whole isn't contradictory. Consider the case where A is denied (indeed the statement implies not-A). So, think about mathematical proofs of the sort where we say something like "if A is true then B must be odd and not-odd." A number can't be odd and not odd so A must be false. -
 bongo fury
1.7kUnless A is already a contradiction, e.g. defined as C ∧ ~C. Then, regardless of whether A is affirmed or denied, both (A entails B) and (A entails notB) are true. And neither one contradicts the other.
bongo fury
1.7kUnless A is already a contradiction, e.g. defined as C ∧ ~C. Then, regardless of whether A is affirmed or denied, both (A entails B) and (A entails notB) are true. And neither one contradicts the other. -
 javra
2.7kI think issues like the cat are simply mistranslations and over simplifications. The statement should be something like:
javra
2.7kI think issues like the cat are simply mistranslations and over simplifications. The statement should be something like:
The cat is sitting across the threshold of the house, therefore some of the cat is in the house and some of the cat is in the house. — Count Timothy von Icarus
I don't findA = a cat is sleeping outstretched on the threshold of the entry door to a house. — javra
to be physically impossible. With a quick whimsical online search, found this pic: https://www.instagram.com/atchoumthecat/p/C5ddrTwLzR6/
In this one pic, the 4-month-old cat's tail is partly inside and some of its whiskers are outside, and the threshold is to a sliding door rather than an entry door, but I think it amply illustrates my point: given a sufficiently wide threshold and a sufficiently small cat, A as I described it can in fact obtain. This as a real-world example. -
 javra
2.7kDo (A entails B) and (A entails notB) contradict each other? — bongo fury
javra
2.7kDo (A entails B) and (A entails notB) contradict each other? — bongo fury
Only if (A entails B) and (A entails notB) occur in the exact same respect (and, obviously, at the same time), which I find is most often the case. -
 Janus
16.8kI think your basic intuition is correct. It resists the crucial methodological error of "trusting the logic machine to the extent that we have no way of knowing when it is working and when it is not" (↪Leontiskos). We need to be able and willing to question the logic tools that we have built. If the tools do not fit reality, that's a problem with the tools, not with reality (↪Janus). — Leontiskos
Janus
16.8kI think your basic intuition is correct. It resists the crucial methodological error of "trusting the logic machine to the extent that we have no way of knowing when it is working and when it is not" (↪Leontiskos). We need to be able and willing to question the logic tools that we have built. If the tools do not fit reality, that's a problem with the tools, not with reality (↪Janus). — Leontiskos
I agree, and if formal logic contradicts the logic inherent in our ordinary ways of speaking and making claims about things, I can't see the fault laying with ordinary parlance.
However, reading (A implies notB) as "something other than B (caveat: also) follows from A". would be consistent with "B follows from A", because it would not deny that B also follows from A.
— Janus
Yeah that's a good explanation for why it intuitively makes sense that they're a contradiction. — flannel jesus
Actually I had thought that it was an explanation for why, on that interpretation, it makes sense that they are not a contradiction, while maintaining that on the other reading it seems to makes no sense to claim that they are not a contradiction.
Consider this as an intuitive explanation for why they aren't a contradiction:
A implies B can be rephrased as (not A or B)
A implies not B can be rephrased as (not A or not B)
Do you think (not A or B) and (not A or not B) contradict?
I read 'A implies B' as (if A then B) or (not (A and notB)). It's a fair while since I studied predicate logic, though.
Only if (A entails B) and (A entails notB) occur in the exact same respect (and, obviously, at the same time), which I find is most often the case. — javra
Is it not a given that we should understand A and B to refer to the same things in both? -
 TonesInDeepFreeze
3.8kIf the long reply made you feel better, that's fine. — Philosophim
TonesInDeepFreeze
3.8kIf the long reply made you feel better, that's fine. — Philosophim
So many things wrong packed into just that one sentence. (1) My post was hardly that long. (2) It's length was a function of the explanation it contains. (2) I don't begrudge posters making posts at any length they want. (3) What is the purpose of mentioning length if not as wedge to discredit? (4) The post carries a lot more message than being a way to "feel better". (5) You did not even address the points I made, but instead you tried to dismiss it with innuendo (if not outright implication) that the post is just a bunch of me trying to fell better.
You can't argue against how you come across to other people on a forum. — Philosophim
(1) I can't dispute that certain people feel certain ways. (2) I can dispute people's representations and characterizations of my posts and my interior, including feelings. (3) How you feel about me does not represent how all others feel about me. (4) And likewise, you can't argue against how you come across to me.
Hopefully we'll have a better encounter in another thread. — Philosophim
Hopefully so. And hopefully in this thread. The odds of that happening would be greatly improved by not sending your first post to me to include "Don't be a troll".
Good luck in explaining your side, I do agree with it. — Philosophim
Don't need luck. I've been explaining my thoughts well. I am glad for your agreement.
you're running into a mismatch between most people's general sense of seeing -> as a strict conditional. — Philosophim
(1) '->' is a symbol ordinarily used for material implication; while the strict conditional usually uses a different symbol or is written with '->' and a modal operator.
(2) Strict implication is what might be in mind in certain everyday contexts, but my guess is that relevance is crucial in average everyday contexts. If A is not relevant to B, then I bet most people would just take "if A then B" and "Necessarily if A then B" to be nonsense and, while some people would take it as false on account of being nonsense, others would just say it is plain nonsense.
(2) I have said at least a few times that I quite understand that there are many other notions of 'implies' other than material implication. There is relevance logic, strict implication, and I would bet there are other formal and/or philosophical approaches. And there ar everyday notions that may run from deliberate to not even having a worked out notion of what 'implies', especially to extent of untangling the original thread question (I would guess that you could ask many people walking around the original thread question and their best answer would be "huh?").
in your field or life 'material conditional' is a common phrase, but for most people who use logic, this is never introduced. For them, it's almost always seen as a strict conditional. Remember that this forum is populated by all types of people, and most of them are not logicians or philosophers themselves. — Philosophim
In a philosophy forum, in its 'Logic and Philosophy of Mathematics' section, a question posed that would hardly ever occur in everyday discourse, may be answered however one likes to answer it. Since in logic, the overwhelmingly most common sense is the material conditional, I answered in that context. I do not disallow anyone from answering in other contexts. (I did say that my answer is 'that simple" and regardless "digressions". But I also posted that I do not claim that material implication is the only context that can be countenanced).
Explaining and contrasting a strict conditional vs a material conditional should make the issue clear for most people. — Philosophim
That's fine. No one is stopping you from posting your explanation and contrast. But also, a hearty explanation would include other notions too, especially relevance. -
 TonesInDeepFreeze
3.8kI have no issue with being corrected or told new things. — Philosophim
TonesInDeepFreeze
3.8kI have no issue with being corrected or told new things. — Philosophim
Note that my corrections did not presuppose that only material implication can be countenanced.
he jumped into a conversation I was having with another poster without context — Philosophim
Oh please, everyone enters a thread by "jumping in" in media res. Maybe you noticed that there aren't 'hand waving' icons to click to be recognized like in a video meeting. And there are no "having a conversation with another poster" that doesn't admit of anyone "jumping in" to comment. And "out of context" would mean that my remarks were misleading or not worthwhile on account of them needing to be modified by context. That was not the case with my remarks.
and when I asked him to clarify his issue he came across as dismissive. — Philosophim
You didn't just ask me to clarify. You insulted personally with "Don't be a troll", and I did provide you with what you requested.
I encourage you not to do the same and jump into another conversation between two people. — Philosophim
I encourage anyone to jump into any conversation, among any posters, as much as they like.
You don't have an exclusive right to be the only one commenting on what other posters say, including what they say to you.
/
And as preemptory in case of complaints that I should just "let it go" with you, note that you are publicly making faulting me as a poster, not just as to what I've said on the topic but on a personal basis also. It is quite proper that I defend myself. I don't have to just let you run your posts running me down unanswered. -
 javra
2.7kIs it not a given that we should understand A and B to refer to the same things in both? — Janus
javra
2.7kIs it not a given that we should understand A and B to refer to the same things in both? — Janus
No. We presume this to be so most of the time for good reason, but it is not a universal given.
Consider: the metaphysical understanding of reality, R, entails both that a) there is a self and b) there is no self.
If the entailment here referred to in regard to both (a) and (b) occurs in the exact same respect (to include relative to the exact same metaphysical or else philosophical perspective concerning the exact same reality/actuality - this even if various levels of reality/actuality were to be implicitly endorsed, e.g. the mundane physical reality of maya and the ultimate reality of literal nonduality), then it would be a flagrant contradiction and thereby a necessarily false proposition. One can of course argue that this is in fact the case, but one cannot thereby establish that this is what the speaker in fact held in mind and thereby intended to express. (Example: the speaker might have intended that in the mundane physical reality of maya there of course occurs the reality of selfhood, this just as much as that in the ultimate reality of literal nonduality no such thing as selfhood is possible - with both these affirmed truths being equally entailed by the very same R at the same time but in the different respects just mentioned.)
Welcome to The Philosophy Forum!
Get involved in philosophical discussions about knowledge, truth, language, consciousness, science, politics, religion, logic and mathematics, art, history, and lots more. No ads, no clutter, and very little agreement — just fascinating conversations.
Categories
- Guest category
- The Lounge
- General Philosophy
- Metaphysics & Epistemology
- Philosophy of Mind
- Ethics
- Political Philosophy
- Philosophy of Art
- Logic & Philosophy of Mathematics
- Philosophy of Religion
- Philosophy of Science
- Philosophy of Language
- Interesting Stuff
- Politics and Current Affairs
- Humanities and Social Sciences
- Science and Technology
- Non-English Discussion
- German Discussion
- Spanish Discussion
- Learning Centre
- Resources
- Books and Papers
- Reading groups
- Questions
- Guest Speakers
- David Pearce
- Massimo Pigliucci
- Debates
- Debate Proposals
- Debate Discussion
- Feedback
- Article submissions
- About TPF
- Help
More Discussions
- Panpsychism implies that computers are sacred expressions of human potential
- Why special relativity does not contradict with general philosophy?
- What's up with people who contradict themselves on their own sincerity & can't see their own faults?
- How "free will is an illusion" does not contradict theology
- Other sites we like
- Social media
- Terms of Service
- Sign In
- Created with PlushForums
- © 2025 The Philosophy Forum









