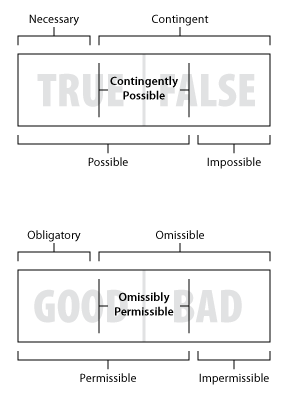
-
 mads
1Hello everyone.
mads
1Hello everyone.
I would like to ask if, in terms of truth, do we only have true or false, zero or one, yes or no, or does exist something else in the middle describing something between the two. Should a thing lik this exist, what would it mean for our language, for mathematics, for computing, for human relationships? -
 fishfry
3.4kQuantum computers are based on such an idea. In normal probability theory you take 1 and 0 to be certainty and impossibility, and a probability is a real number between them. In quantum theory, a probabilty amplitude is a complex number (that is, of the form a + bi where ); and the square of its length is the probability that some event will be observed. They use this to implement qubits, which are bits that are neither 1 nor 0 until they are, or some such popularized explanation.
fishfry
3.4kQuantum computers are based on such an idea. In normal probability theory you take 1 and 0 to be certainty and impossibility, and a probability is a real number between them. In quantum theory, a probabilty amplitude is a complex number (that is, of the form a + bi where ); and the square of its length is the probability that some event will be observed. They use this to implement qubits, which are bits that are neither 1 nor 0 until they are, or some such popularized explanation. -
 Wayfarer
23.7kBy and large, Kaccayana, this world is supported by a polarity, that of existence and non-existence. But when one sees the origination of the world as it actually is with right discernment, "non-existence" with reference to the world does not occur to one. When one sees the cessation of the world as it actually is with right discernment, "existence" with reference to the world does not occur to one. — The Buddha
Wayfarer
23.7kBy and large, Kaccayana, this world is supported by a polarity, that of existence and non-existence. But when one sees the origination of the world as it actually is with right discernment, "non-existence" with reference to the world does not occur to one. When one sees the cessation of the world as it actually is with right discernment, "existence" with reference to the world does not occur to one. — The Buddha
Kaccayanagotta Sutta
See also https://en.wikipedia.org/wiki/Two_truths_doctrine -
 Andrew M
1.6kI would like to ask if, in terms of truth, do we only have true or false, zero or one, yes or no, or does exist something else in the middle describing something between the two. — mads
Andrew M
1.6kI would like to ask if, in terms of truth, do we only have true or false, zero or one, yes or no, or does exist something else in the middle describing something between the two. — mads
I think as a general rule, the Law of Excluded Middle applies. But there can be exceptions, e.g., fuzzy logic, or loaded questions like "Have you stopped beating your wife?" -
 Pfhorrest
4.6kThere are also paraconsistent and intuitionistic logics that can deal with propositions being either (respectively) both true and false, or neither true and false. (Alternate links from Stanford Encyclopedia of Philosophy: paraconsistent logic and intuitionistic logic).
Pfhorrest
4.6kThere are also paraconsistent and intuitionistic logics that can deal with propositions being either (respectively) both true and false, or neither true and false. (Alternate links from Stanford Encyclopedia of Philosophy: paraconsistent logic and intuitionistic logic). -
 javra
2.7k
javra
2.7k
@fishfry nicely addressed the dichotomy between zero and one.
As to "yes" and "no" there is "maybe". Example: "Will you be going to the event?"; here "yes" (I am decided on going), "no" (I am decided on not going), and the intermediate of "maybe" (I have not yet decided whether or not I will go) call all make sense.
As to true and false, there can be propositionally expressed partial truths which are thereby incomplete and, in so being, can at least given the epistemic impression of being partially false. A relatively easy, but trite, example is most any honest answer to the question of "what do you see?" That one is focusing one's visual attention on some item, say a lamp, is true. But it is equally true that one also sees a plethora of other items while focusing one's visual attention on the lamp - for instance, that on which the lamp rests and the immediate background to the lamp. So, at least form one semantic angle, the answer of "I'm seeing a lamp" will be a partial truth - also being partially false in not conveying all the other givens that are likewise seen by me simultaneously. Here, no deception is intended - but one cannot help but give a partial truth to the question. Hence, if you ask me what I see, I reply "a rock", and you then interpret by this that I don't see the mountain behind the rock, I wouldn't have lied to you about what I do and don't see - even though I also saw the mountain in the background.
BTW, I so far find that the Law of Excluded Middle always applies to cases where the alternatives are at least interpreted to be clearly dichotomized. The cat is either inside the room or outside the room - with no middle ground possible in this clear dichotomy between "inside" and "outside". But change the contextual semantics one interprets and one can change the possibilities addressed, thereby changing the parameters of what is intended by some given statement. For example, to answer that "the cat is both inside and outside the room" can make sense without negating either the Law of Excluded Middle or the Law of Noncontradiction, from which the former is derived - this by interpreting the cat to be sitting on the threshold of the given room's door. Here, the state of affairs of the cat is to be both inside and outside the room or, else, neither inside nor outside the room, in perfectly noncontradictory manners.
Hence, imo, all apparent contradictions are either contradictory and thereby nonsense or, else, can be interpreted to be partial truths. The statement, "they're the same but different" serves as an example: it either intends "same" and "not the same" at the same time and in the same respect as a middle between the two - in which case its nonsense - or that they're the same in one way and not the same in a different way at the same time - in which case it's use is intended to convey a noncontradictory state of affairs without in any way negating the Law of the Excluded Middle. Just that it does this without explicitly stating what the addressed, complete state of affairs is, and so can be interpreted as an expressed partial truth.
Same then applies to statements such as "neither is there a self nor is there not a self": these are either nonsense due to being contradictory and thereby breaking with the Law of the Excluded Middle or, else, convey more complex and noncontradictory states of affair by expressing partial truths. Hence, as with the cat being neither inside nor outside the room, but in-between on the door's threshold, were these statements to be nonconctradictory, they then would not break with the Law of the Excluded Middle.
So:
I would like to ask if, in terms of truth, do we only have true or false, zero or one, yes or no, or does exist something else in the middle describing something between the two. — mads
In my opinion, givens can occur in the middle of these conceptual dichotomies, but, when they do so occur, they will yet manifest in manners accordant to the Law of Noncontradicition and the Law of the Excluded Middle. -
 tim wood
9.4kthe cat to be sitting on the threshold of the given room's door. — javra
tim wood
9.4kthe cat to be sitting on the threshold of the given room's door. — javra
Always between indoors and outdoors while the cat considers and takes counsel with itself whether, when all is said and done, it really wishes to be inside or outside. And this most often on Winter days. By the way, when did you meet my cat? -
 Pfhorrest
4.6kLaw of Excluded Middle or the Law of Noncontradiction, from which the former is derived — javra
Pfhorrest
4.6kLaw of Excluded Middle or the Law of Noncontradiction, from which the former is derived — javra
Not quite. The two together comprise the Law of Bivalence. Excluded middle says it has to be either true OR false. Non-contradiction says it must not be both true AND false. Together they make up Bivalence: true XOR false. -
TheMadFool
13.8kWhat is clear is that when we make a statement, any statement, without any qualification, it is always the case that truth is implied/implicit. Take the following statements into consideration:
1. One is greater than zero
2. There's a 90% chance of rain tomorrow
3. Aliens may have visited earth in the past
Statement 1 has the mark of certainty, there are no words in it suggesting hesitation as to its truth. So, it's either true or false depending on whether it's got a sound argument to back it up or not. Statement fully unpacked is 1a. One is greater than zero is true
Statement 2 is clearly probabilistic in character (90% chance) and while the event (rainfall) isn't completely certain to occur, the statement itself (2) is implied to be true. It could be false if the number crunching that led to 90% had errors. Whatever the case, statement 2 is either true or false and not something in between. Statement 2 fully unpacked is 2a. There's a 90% chance of rain tomorrow is true
Statement 3 is also probabilistic but doesn't have a number assigned to the probability of aliens having visited the earth in the past. Nevertheless, it's implied that statement 3 is true while it's possible for it to be false. There's no middle ground here between true and false. Statement 3 unpacked is 3a. Aliens may have visited the earth in the past is true.
As you can see, even statements that deal with uncertain stuff are implicitly claimed as true despite the possibility of them being false. There is nothing in-between betwixt true and false.
That said, there are certain statements like:
4. Aliens had visited earth in the past
At first glance proposition 4 isn't true or false but 4 can easily be rephrased, with no loss in meaning at all, as:
4a. Aliens may have visited the earth in the past
Statement 4a is either true or false.
I guess we have to keep in mind the difference between probabilistic phenomena (uncertain) and propositions about probabilistic phenomena (certain truth/falsity).
My two cents. -
 javra
2.7k
javra
2.7k
What then do you make of this:
The law of excluded middle is logically equivalent to the law of noncontradiction by De Morgan's laws [...] — https://en.wikipedia.org/wiki/Law_of_excluded_middle
And this:
The [law of excluded middle] should not be confused with the semantical principle of bivalence, which states that every proposition is either true or false. — https://en.wikipedia.org/wiki/Law_of_excluded_middle
I know, it's Wikipedia. Still... -
 Pfhorrest
4.6kFor that first quote: Given classical (bivalent) logic, the excluded middle and non-contradiction are equivalent by de Morgan's laws. But if you deny either of them, you're leaving classical logic, in which case you could deny just one or the other of them, and not both. Intuitionistic logic denies the excluded middle but not non-contradiction (something can be neither true nor false). Paraconsistent logics deny non-contradiction but not the excluded middle (something can be both true and false).
Pfhorrest
4.6kFor that first quote: Given classical (bivalent) logic, the excluded middle and non-contradiction are equivalent by de Morgan's laws. But if you deny either of them, you're leaving classical logic, in which case you could deny just one or the other of them, and not both. Intuitionistic logic denies the excluded middle but not non-contradiction (something can be neither true nor false). Paraconsistent logics deny non-contradiction but not the excluded middle (something can be both true and false).
And for the second quote: That's completely correct, since the excluded middle is not, by itself, identical to bivalence. You need both excluded middle ("either T or F") and non-contradiction ("not both T and F") to make up bivalence ("T xor F"). -
 javra
2.7kI acknowledge that argument/observation. Thanks for the correction.
javra
2.7kI acknowledge that argument/observation. Thanks for the correction.
So back to the LEM not being derived from the LNC. Having mulled it over some, should have said the LEM is derived from the Law of Identity (LID) via notions obtained from the LNC – with the LNC being derived from the LID. For instance: If A is A (ID) then A cannot both be A and not-A at the same time and in the same respect (NC); next, if the LID and the LNC, neither can A be something intermediate between A and not-A which, thereby, would be neither A nor not-A.
Any objections to that formulation? For the record, I don’t know of any non-arbitrary way to obtain the LEM in manners not derived from, else dependent on, the LID via notions of “A and not-A” provided in the LNC. If you happen to, curious to learn of them.
As an aside: Dialetheism to me … well, let’s say doesn’t exist on the very grounds that it does. -
 Banno
26.3kI would like to ask if, in terms of truth, do we only have true or false, zero or one, yes or no, or does exist something else in the middle describing something between the two. — mads
Banno
26.3kI would like to ask if, in terms of truth, do we only have true or false, zero or one, yes or no, or does exist something else in the middle describing something between the two. — mads
While there are variations in logic which do not rely on bivalence - being true, or false, with nothing in between - standard bivalent logic has long been preferred, and gets us further. -
 Gnomon
3.9k
Gnomon
3.9k
Yes, in mathematics, it's called "Probability", or "Fuzzy Logic". In philosophy it's called "the Excluded Middle" of a continuum, and in ordinary parlance it's called "Maybe".. Only if the postulates are directly contradictory, is there no middle ground. But that kind of certainty is hard to come by. Which is why philosophers argue a lot. :smile:I would like to ask if, in terms of truth, do we only have true or false, zero or one, yes or no, or does exist something else in the middle describing something between the two. — mads -
 aletheist
1.5k
aletheist
1.5k
Yes, I find it misleading because it employs only one variable (A) rather than distinguishing between a subject (S) and a predicate (P) as attributed to it by a proposition. I also prefer referring to the so-called "laws of thought" as principles, since (as already noted) there are viable logical systems in which they do not hold.If A is A (ID) then A cannot both be A and not-A at the same time and in the same respect (NC); next, if the LID and the LNC, neither can A be something intermediate between A and not-A which, thereby, would be neither A nor not-A. Any objections to that formulation? — javra
The principle of contradiction (PC) is that S is not both P and not-P at the same time and in the same respect; i.e., it is never the case that both "S is P" and "S is not-P" are true. The principle of excluded middle (PEM) is that S is either P or not-P at any given time; i.e., it is always the case that either "S is P" or "S is not-P" is true. Neither of these entails the other, but taken together they entail the principle of bivalence (PB) along with the rule of double negation--if S is not not-P, then S is P. -
 3017amen
3.1k
3017amen
3.1k
In a word, yes. Examples include violations of LEM/bivalence (principle of Vagueness/ambiguity), consciousness (consciousness, sub-consciousness and unconsciousness), Being and Becoming, self referential statements (unresolved paradox/incompleteness), etc..
Living this life is indeed a mystery and somewhat incomplete and irrational and/or transcends the axioms of formal logic :snicker:
(Think of it another way; the metaphorical cosmic computer operates from yes/no and either/or (binary) systems, but living life isn't like that. If it were, we wouldn't survive meltdowns- well some people don't survive them anyway!)
Welcome to The Philosophy Forum!
Get involved in philosophical discussions about knowledge, truth, language, consciousness, science, politics, religion, logic and mathematics, art, history, and lots more. No ads, no clutter, and very little agreement — just fascinating conversations.
Categories
- Guest category
- The Lounge
- General Philosophy
- Metaphysics & Epistemology
- Philosophy of Mind
- Ethics
- Political Philosophy
- Philosophy of Art
- Logic & Philosophy of Mathematics
- Philosophy of Religion
- Philosophy of Science
- Philosophy of Language
- Interesting Stuff
- Politics and Current Affairs
- Humanities and Social Sciences
- Science and Technology
- Non-English Discussion
- German Discussion
- Spanish Discussion
- Learning Centre
- Resources
- Books and Papers
- Reading groups
- Questions
- Guest Speakers
- David Pearce
- Massimo Pigliucci
- Debates
- Debate Proposals
- Debate Discussion
- Feedback
- Article submissions
- About TPF
- Help
More Discussions
- Other sites we like
- Social media
- Terms of Service
- Sign In
- Created with PlushForums
- © 2025 The Philosophy Forum