-
 Abdul
46Let's say in a certain country that 25,000 people are needed for a certain presidential candidate to win. 24,999 votes in favor of this candidate are already cast. One guy says to himself, "Hmph...I'm only one guy. My vote doesn't matter."
Abdul
46Let's say in a certain country that 25,000 people are needed for a certain presidential candidate to win. 24,999 votes in favor of this candidate are already cast. One guy says to himself, "Hmph...I'm only one guy. My vote doesn't matter."
Now that's all well and good. You could easily find someone else to take his place. However, other people begin to think likewise and the 25,000 goal is never met. At what point does this mindset become destructive enough to create an unintended social effect?
At what threshold does a change in individual behavior cause a major unintended social effect? -
 tim wood
9.5k
tim wood
9.5k
It must seem like a math/statistics question. It isn't. It makes a difference when even one opts out.At what point does this mindset become destructive enough to create an unintended social effect?
At what threshold does a change in individual behavior cause a major unintended social effect? — Abdul -
 T Clark
14.3kAt what threshold does a change in individual behavior cause a major unintended social effect? — Abdul
T Clark
14.3kAt what threshold does a change in individual behavior cause a major unintended social effect? — Abdul
In the United States in 2000, George Bush won Florida by fewer than 600 votes out of 6 million. That lead to him being elected President, which lead to the war in Iraq, the death of 4,000 Americans and an estimated 500,000 others. It also lead to ISIS and the migration of a million Syrians and others to Europe which has undermined governments and social conditions. -
 BC
13.7kYour 25,000th voter doesn't know what the total is, of course. For all he knows, the election IS already decided by many votes. And even if the vote was all but a tie, everyone who voted (either way) matters as much as the last one to vote.
BC
13.7kYour 25,000th voter doesn't know what the total is, of course. For all he knows, the election IS already decided by many votes. And even if the vote was all but a tie, everyone who voted (either way) matters as much as the last one to vote.
At what threshold does a change in individual behavior cause a major unintended social effect? — Abdul
Behavior changed from what?
Let's say 3000 people are watching a broadway play premier. No one has seen the play before. The expected behavior is that everyone will remain seated until the intermission. Everyone knows is expected, because it is well established social convention.
Suppose, during the first act that 25 people get up and exit the theater at various times. Has an unintended social effect occurred? Then, suppose that during the second act (before the intermission) 30 more people scattered throughout the theater get up and exit the theater. 55 people have now walked out.
My guess is that at some point during the first two acts people walking out will have had a social effect (intended or not) on the 2945 remaining audience and the cast. Probably somebody has studied this. Is there a difference between one person walking out about every minute or people walking out at irregular intervals.
If people walk out of a production, does that change the opinions about the play of the people who stayed? I would guess it does (favorably or negatively).
Life, including politics presents a myriad instances where the uncoordinated actions of individuals can have significant social effects. In open voting (show of hands) the act of voting puts pressure (positive or negative) on others in the room. In legislative voting where votes are displayed on an electronic board, it is clear that some legislators are measuring the vote before they cast their own vote. Sometimes votes are changed.
In a public speaking engagement, one or two loud objectors can create significant problems for the speaker; if the speaker cannot dominate the audience, then he may lose his audience, especially if additional objectors pop up. -
 WISDOMfromPO-MO
753Your 25,000th voter doesn't know what the total is, of course. For all he knows, the election IS already decided by many votes. — Bitter Crank
WISDOMfromPO-MO
753Your 25,000th voter doesn't know what the total is, of course. For all he knows, the election IS already decided by many votes. — Bitter Crank
Or he is in, oh, Oregon, the polls have closed in the Eastern time zone where most voters are, news outlets have already projected a winner in most states, so he figures that the election is already mostly decided and his late vote won't matter. -
 T Clark
14.3kSounds related to the butterfly effect. Proves how powerful seemingly simple decisions are.
T Clark
14.3kSounds related to the butterfly effect. Proves how powerful seemingly simple decisions are.
On a side note, do you think the butterfly effect is related in any way to determinism? — Abdul
The butterfly effect is one of those subjects, like quantum mechanics, which people don't understand and misinterpret because the concept seems to have metaphorical power. Also, my understanding is not deep. For that reason, I try to be careful while discussing it and similar subjects - chaos theory, complexity, emergence. The events in the Florida election are different. At least the way I presented it in my post, the chain of events was causal - billiard balls. The butterfly effect is something different. It reflects events for which no causal connection can be identified, if my understanding is correct, even in theory.
Whoever comes to correct my explanation, please be kind.
@fdrake- I've read a bit about chaos theory and it seemed to me to be almost a kind of statistics - strange attractors and such. I don't know if you have any experience with this subject. If you do, do you know what I mean? -
 gurugeorge
514At what threshold does a change in individual behavior cause a major unintended social effect? — Abdul
gurugeorge
514At what threshold does a change in individual behavior cause a major unintended social effect? — Abdul
It has a continuous effect right now, and always, in democracies, it's called "rational ignorance."
Compare and contrast with buying a car. Deliberation prior to buying a car leads to getting the car you decide to buy, in a way that deliberation prior to a political decision doesn't. Therefore, for most people, it doesn't pay to be informed.
This is why politics is shallow, prone to demagoguery, easily manipulable by rhetoric and the influence of mere charisma, etc. With democracy most of the time, people just go with their gut on who seems like a decent enough chap or chapess, and hope for the best.
However, while this applies to large scale democracy at the national or federal level, it's different the more localized the democratic process is. When it's a local election where everyone knows each others' asses, and the people up for election are known quantities in their local area (here a known local teacher, there a known local businessman), there's more of a chance of an informed decision being made because less of a special effort with a great cost has to be made to be informed.
Another consequence of rational ignorance is the tendency of democracies to become managerially run ("Deep State", etc.). The problems are so complex and difficult, and often have temporal ramifications that don't oblige 4 year periodicity, that they're better run by "experts" anyway, and it's easier to manipulate public opinion and lead it (so that voters are rhetorically tricked into rubberstamping decisions already long-since made in smoke-filled rooms, so to speak), than to follow it.
But the problem with this is that it depends on the type of "expert." Politics run by statesmen who have noble intentions and a feel (or knack) for politics borne of experience, is all well and good, politics run by bureaucracy where decisions are made on the basis of shallow intellectual analysis (particularly based on statistics that might not even be carving nature at the joints anyway) can be terrible - and tend to a tyranny of mediocrity. (This would be comparable to business: a business run by people who have a proven track record, and just happen to have whatever neat trick in the brain is necessary to do successful stuff, whatever that knack may be, is better than a business run like a machine on pseudo-scientific principles. Or again, one thinks of those awful agricultural or city-design experiments of the 20th century, which often overrode local or traditional wisdom, with disastrous results. In reality, you do need a bit of a blend of the two - brains with neat tricks and experience/tradition, plus some scientific analysis and managerial efficiency - but the buck should stop with the brain that has the neat trick.) -
 fdrake
7k
fdrake
7k
I had nothing to do this evening, so:
Big difference between chaotic systems and stochastic systems. Chaotic systems are typically (as a matter of definition) deterministic, chaos occurs when small changes in initial conditions produce big changes in long term dynamics. A dynamical system, mathematically, is formed by the repeated application of a function to a set of initial conditions (numbers).
The classical example is the logistic map, which is defined by:
Imagine . And set as the starting point.
(this code works in the open-source programming language/statistical software R)logisticmap=function(x0,n,r){ xseq=rep(0,n) xseq[1]=x0 for(i in 2:n){ xseq[i]=r*xseq[i-1]*(1-xseq[i-1]) } return(xseq) }
this takes a number and spits out a number , then it takes and spits out a number ...You can go on forever.
Finding the 10th term of the sequence with that is...
that's about -6000.
Now I'm gonna subtract 0.0002 from and see how the system evolves, it has tenth term:
that's just under +1.
Four orders of magnitude different, and of opposite sign. The situation gets worse when you take more terms. This sensitivity to initial conditions is what characterises (deterministic) chaos.
Certain functions have interesting patterns in them, it's possible to 'get stuck' in a repeating sequence or part of the set of possible values for the system. The places you get stuck in are called attractors. The classical example of this is the Mandlebrot set.
The Mandlbrot set is the set of all points in the complex plane that don't escape to infinity under the map:
This set of functions will let you generate approximations to the Mandlebrot set in R:
znorm=function(complexno){sqrt((Re(complexno))^2+(Im(complexno))^2)} mandlebrot=function(c,n){ k=1 z=0 thenorm=0 while(k<n && is.finite(thenorm)){ z=z^2+c thenorm=znorm(z) k=k+1 } return(is.finite(thenorm)) } mandlevec=Vectorize(mandlebrot,vectorize.args = "c") cs=seq(-2,2,by=0.01) cs=expand.grid(cs,cs) complexes=complex(real=cs[,1],imaginary = cs[,2]) truefalse=mandlevec(c=complexes,n=100) xs=cs[,1][truefalse] ys=cs[,2][truefalse] points(xs,ys)
which gives you the pretty picture:
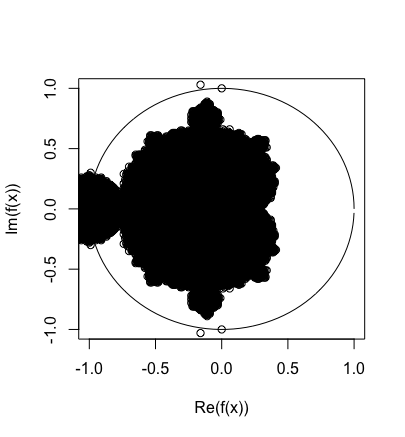
of the central cardioid of the Mandlebrot set inside the unit circle.
The Mandlebrot set (strictly speaking) is not a strange attractor - but it gives off the intuition of one. A complicated fractal structure implicit in the geometry of a dynamical system. The attractor is a set of points that don't shoot off to infinity, and it's called a strange attractor if the attractor is itself a fractal.
Stochastic systems, by contrast, are driven by random fluctuations - their dynamics are random in nature, how they 'realise themselves' is random, rather than consisting of specific, determinate mappings. I don't know much about their attractors etc, but there's a similar canonical example in Brownian motion.
A really simple stochastic system to visualise is balancing a small perfectly symmetrical infinitely thin piece of paper on the head of an infinitely thin pin. The motion of the air particles around it causes it to topple in some random direction. IE, the force which drives the dynamics, mapping state to the next state, evolves as a random variable. From what little experience I have with stochastic systems (their differential equations) I can say this: there be fucking dragons. -
 fdrake
7k
fdrake
7k
That's how weather prediction works. People fit complicated models of atmospheric pressure changes, correlate it to statistical summaries of current temperatures and evolve the system forward in time from a range of initial conditions (similar things to the current temperature/pressure measurements, elevation, distance from the sea etc), so when they say '80% chance of rain' - they mean '80% of the models we ran had rainfall in this area'! -
 LD Saunders
312Well, aren't you assuming that this is a bad thing when there is no basis for saying so? After all, if a particular candidate loses do to low voter turnout, that may or may not be a bad thing.
LD Saunders
312Well, aren't you assuming that this is a bad thing when there is no basis for saying so? After all, if a particular candidate loses do to low voter turnout, that may or may not be a bad thing.
Welcome to The Philosophy Forum!
Get involved in philosophical discussions about knowledge, truth, language, consciousness, science, politics, religion, logic and mathematics, art, history, and lots more. No ads, no clutter, and very little agreement — just fascinating conversations.
Categories
- Guest category
- The Lounge
- General Philosophy
- Metaphysics & Epistemology
- Philosophy of Mind
- Ethics
- Political Philosophy
- Philosophy of Art
- Logic & Philosophy of Mathematics
- Philosophy of Religion
- Philosophy of Science
- Philosophy of Language
- Interesting Stuff
- Politics and Current Affairs
- Humanities and Social Sciences
- Science and Technology
- Non-English Discussion
- German Discussion
- Spanish Discussion
- Learning Centre
- Resources
- Books and Papers
- Reading groups
- Questions
- Guest Speakers
- David Pearce
- Massimo Pigliucci
- Debates
- Debate Proposals
- Debate Discussion
- Feedback
- Article submissions
- About TPF
- Help
More Discussions
- Other sites we like
- Social media
- Terms of Service
- Sign In
- Created with PlushForums
- © 2025 The Philosophy Forum









